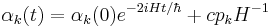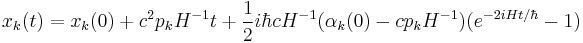Zitterbewegung
Zitterbewegung (English: "trembling motion", from German) is a theoretical rapid motion of elementary particles, in particular electrons, that obey the Dirac equation. The existence of such motion was first proposed by Erwin Schrödinger in 1930 as a result of his analysis of the wave packet solutions of the Dirac equation for relativistic electrons in free space, in which an interference between positive and negative energy states produces what appears to be a fluctuation (at the speed of light) of the position of an electron around the median, with a circular frequency of 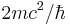 , or approximately 1.6×1021 Hz.
, or approximately 1.6×1021 Hz.
Zitterbewegung of a free relativistic particle has never been observed, but the behavior of such a particle has been simulated with a trapped ion, by putting it in an environment such that the non-relativistic Schrödinger equation for the ion has the same mathematical form as the Dirac equation (although the physical situation is different). [1] [2]
Contents |
Theory
The time-dependent Schrödinger equation
where 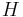 is the Dirac Hamiltonian for an electron in free space
is the Dirac Hamiltonian for an electron in free space
in the Heisenberg picture implies that any operator Q obeys the equation
In particular, the time-dependence of the position operator is given by
where 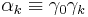 .
.
The above equation shows that the operator 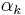 can be interpreted as the kth component of a "velocity operator".
can be interpreted as the kth component of a "velocity operator".
The time-dependence of the velocity operator is given by
where ![\sigma_{kl} \equiv \frac{i}{2}[\gamma_k,\gamma_l]](/2012-wikipedia_en_all_nopic_01_2012/I/a81e36de2ae61a63e6fb5cafb7b8c479.png) .
.
Now, because both 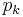 and
and  are time-independent, the above equation can easily be integrated twice to find the explicit time-dependence of the position operator. First:
are time-independent, the above equation can easily be integrated twice to find the explicit time-dependence of the position operator. First:
Then:
where 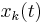 is the position operator at time
is the position operator at time 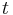 .
.
The resulting expression consists of an initial position, a motion proportional to time, and an unexpected oscillation term with an amplitude equal to the Compton wavelength. That oscillation term is the so-called "Zitterbewegung".
Interestingly, the "Zitterbewegung" term vanishes on taking expectation values for wave-packets that are made up entirely of positive- (or entirely of negative-) energy waves. This can be achieved by taking a Foldy Wouthuysen transformation. Thus, we arrive at the interpretation of the "Zitterbewegung" as being caused by interference between positive- and negative-energy wave components.
See also
- Casimir effect
- Lamb shift
- Stochastic electrodynamics: Zitterbewegung is explained as an interaction of a classical particle with the zero-point field.
References and notes
- ^ "Quantum physics: Trapped ion set to quiver". Nature News and Views. http://www.nature.com/nature/journal/v463/n7277/full/463037a.html.
- ^ Gerritsma; Kirchmair, Zähringer, Solano, Blatt, Roos (2010). Nature. http://www.nature.com/nature/journal/v463/n7277/full/nature08688.html.
Further reading
- E. Schrödinger, Über die kräftefreie Bewegung in der relativistischen Quantenmechanik ("On the free movement in relativistic quantum mechanics"), Berliner Ber., pp. 418–428 (1930); Zur Quantendynamik des Elektrons, Berliner Ber, pp. 63–72 (1931)
- A. Messiah, Quantum Mechanics Volume II, Chapter XX, Section 37, pp. 950–952 (1962)
External links
- The Zitterbewegung Interpretation of Quantum Mechanics, an alternative explanation in addition to positive-negative energy states interference.
- Zitterbewegung in New Scientist
- Geometric Algebra in Quantum Mechanics
- Summary of trapped ion simulation
|
|||||
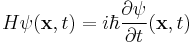
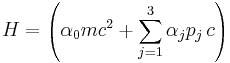
![-i \hbar \frac{\partial Q}{\partial t} (t)= \left[ H , Q \right] \,\!\;.](/2012-wikipedia_en_all_nopic_01_2012/I/3fbaf435b78be451cf7a0044da23970b.png)
![\hbar \frac{\partial x_k}{\partial t} (t)= i\left[ H , x_k \right] = c\alpha_k \,\!\;](/2012-wikipedia_en_all_nopic_01_2012/I/2e4fc75b8a7a8c0aa61dba92ebf4ca4d.png)
![\hbar \frac{\partial \alpha_k}{\partial t} (t)= i\left[ H , \alpha_k \right] = 2[i \gamma_k m - \sigma_{kl}p^l] = 2i[p_k-\alpha_kH] \,\!\;](/2012-wikipedia_en_all_nopic_01_2012/I/3200f5263fd74b3b501a7340887a5367.png)